I have started a research regarding the application of the expression .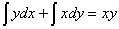
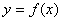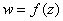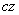First of all, I have started the research by expanding the interpretation of the expression from real number to complex number. Thus, became , and the integral relational expression became , where being a circle on -plane and is expressed as .
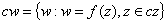If it is a regular function, it is meaningless because 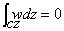will be the result.
There could be something to look for if the function is connected to residue in any way. I have tried to research the various functions connected to residue and characteristics of in terms of various assumed in relation to those functions.
In the research, I have focused on the specificity of 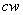in those functions containing . The convergence radius becomes 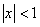, in the case 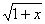is Taylor expanded. In the case 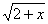, the convergence radius should be . My thinking was that there must some clues in the obstacle created by .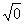
At this point, it came to my attention that "Taylor expansion would be much clearer when thought through in terms of polar coordinates." Hereby, my effort to "apply to Taylor expansion" was completed.

However, my next question was that although the subject became much clearer, how many of those points would really be there?
For example, the cases such as "not even one exists"; "only one exists"; "infinite numbers exist at even intervals like in the case of 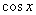" do exist but would there be cases like "only 2 exists" or "only 20 exist". I had a tough time reflecting over these issues. In addition to the aforesaid issues, I also had to be burdened with the issue of how to place limitations on functions.
It just so happened that I had to be hospitalized for three weeks and, because I was bored, I picked up a book on mathematics and as I was gazing through the section covering theory of functions, a certain "workable" theorem grabbed my attention. Through the good use of the said theorem, I was able to complete "Insight with regard to the periodicity of functions".

Japanese sites Mathematical Formulas| Kodawari House| Pinpoint StreetView| Excel VBA Techniques| Excel Formula Analysis|