When my child was still five or six years old, there was a children's learning presentation at a culture hall.
During the idle time, I got to sort of reminisce about my high school mathematics. That was the moment I realized that when integration of a function and integration of its inverse function are thought out in terms of diagrams, it will be the difference in superficial content of a rectangle.
Because I was so excited about this finding, I talked about it to my wife but, of course, since the whole story was Greek to her, she simply let the story pass.
This finding expressed in terms of equation would be .
However, since the relation is such that if 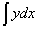is easy to calculate, then, 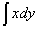would be tough to calculate. Therefore, the compromising of such an addition itself is unnatural. It is quite easy to understand when thought out in terms of diagrams but it is quite difficult to calculate. I was wondering why it was so for about a month.

Japanese sites Mathematical Formulas| Kodawari House| Pinpoint StreetView| Excel VBA Techniques| Excel Formula Analysis|