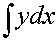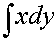
April 1999

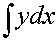Relationship between and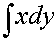

Supposing that 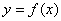is a function defined on the 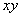plane and bounded variation in closed intervals 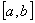, then

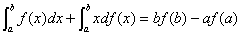
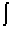( : Lebegue integral )

[Proof]

Refer to the Lebegue-Stielties integral.

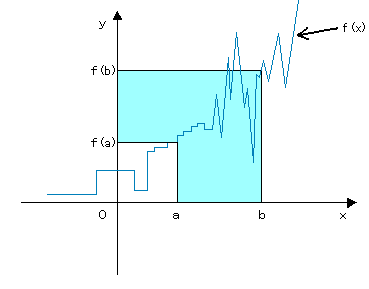
[Interpretation on drawing]

In the drawing shown below, you can see that any function is acceptable if the function is continuous and does not include any point at infinity in the closed intervals .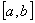

[Postscript]

The formula can be interpreted as a special case of Lebegue-Stielties integral.

Japanese sites Mathematical Formulas| Kodawari House| Pinpoint StreetView| Excel VBA Techniques| Excel Formula Analysis|