


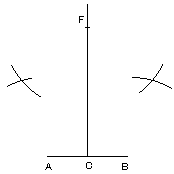
Draw a line segment from point A to point B. That will become an edge of an equilateral pentagon.

Draw an arc having its radius greater than half of the line segment with center at A, draw an arc having the same radius with center at B.
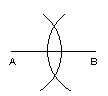
Draw a line passing the two point of intersection obtained above, which line will become the perpendicular to the line.

Now, you use only the upper part of the line.
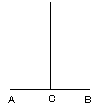
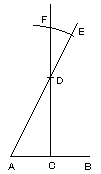
Measure the line segment AB with a compass, and determine a point on the perpendicular from C in order to have the same length as the measurement, which point is named as D.
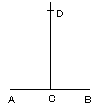
Draw a straight line passing A and D.

Measure the line segment AC with the compass, and determine a point on the line above from D in order to have the same length as the measurement, which point is named as E.
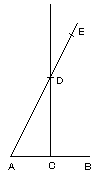
Draw a circle having the radius of a line segment AE placing the compass pin at A. The point of intersection between the circle and the perpendicular is named as F.
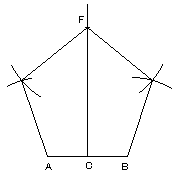
Measure the line segment AB with the compass, and draw circles with the radius of the measurement with centers at A, B, and F.
Draw 4 straight lines each from the two points of intersection obtained above to A and B and F, and you get an equilateral pentagon.
[Other equilateral polygon]
Aside from the equilateral pentagon, you can form equilateral 17- and 257-gons only using a ruler and a compass.
Generally, assuming n as a natural number, if P = 2n + 1 is a prime number, then you can form an equilateral polygon only using a ruler and a compass.
The demonstration of this theorem could be understood if you majored in mathematics in your university or college and can understand group theory.
By the way, I have forgotten it.
[Famous Problem]
It is a mathematical problem proposed in the time of Greek: "Draw a figure only using a ruler and a compass."
1. (Angle-trisecting problem) Divide a given angle into three equal parts.
2. (Cube-doubling problem) Form a cube having the double volume of a given cube.
3. (Circle area problem) Form a square having the same area as a given circle.
These problems are all "impossible" to solve.
Wantzel proved that the problems 1 and 2 are impossible in 1837; Lindemann did for 3 in 1882.
I would like you to understand that it has taken so long a time to put an end to these possibilities.
|
Japanese sites Mathematical Formulas| Kodawari House| Pinpoint StreetView| Excel VBA Techniques| Excel Formula Analysis| |