Mystery of "(-1)x(-1)=1"



[History of number]As extracted from a book on mathematical history
[Decimal system]
Why is a number decimal? It is because we have 10 fingers.
Decimal system has been recorded by ancient people as old as in the times of Egypt and Phoenicia.
Utilizing fingers of one hand, 5 fingers in counting will cause 5-adic system; Utilizing all the fingers of both hands and both feet, 20 fingers will cause 20-adic system.
Some peoples once counted in 5-adic or 20-adic systems, and their low cultural levels caused 5-adic and 20-adic systems to degenerate.
[Fraction]
In Egypt around B.C. 1700, people began to use 1/3, 1/4, and so on due to their necessity to divide in equal parts. However, it is not until around A.D. 300 that they began to use such number as a general number.
[Irrational number]
It is found by an ancient Greek Pythagoras (B.C. 572 - 492), which seems to be describable to the question about "How many times in length is a diagonal of square as large as an edge in his using Pythagorean Theorem?"
[0(zero)]
Zero was created in India. It was symbolized as "E(dot)" at first, "O (circle)" in 870, and afterward "0 (zero)" and introduced to Europe around in 13th century.
To change the subject, currently used so-called "Arabic figure" was originally created in India and yet the Arabic introduced them into Europe, so people began to call them as "Arabic figure."
NOTE: Think about zero as used in notation aside from zero as in -1, 0, 1, 2, - - -. For example, 4056 x 304 is easy to calculate; still, 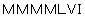 x
x 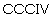 might not be easy due to their difficulty in notation.
might not be easy due to their difficulty in notation.
[Negative number]
Natural numbers and fraction were introduced due to necessity in everyday life; Irrational number due to necessity in geometric requirement. Negative and imaginary numbers are originated from "How to solve algebraic equation."
Around in 1100, secondary equation was found to have its negative root that was yet treated as "nonsense number," "virtual number," or "unreasonable number."
Around in 1600, negative number was the interpreted as "what will represent the opposite direction to a given direction" and recognized as a number.
Above is the history in Europe, and in India "negative number" seems to have been used older than in Europe. Here I stop explaining "negative number," as my historical book is not so extensive.
[Imaginary number]
Imaginary number was first known in 1545 when Caldano (often called as Caldan) solved tertiary equation, but it was treated as "what is merely imaginary" in those days.
Afterwards, people found imaginary number to be very useful, and around in 1850 there was found no one objecting to introduction of it.
As mentioned above, negative and imaginary numbers are quite new comers in mathematical history.
[Explaining (-1) X (-1) = 1]
Multiplication is an application of addition. I will plainly and briefly explain about "(-2) x (-4) " as an example.
In advance, understand that "- (-1)" will result in "1" by representing "the opposite direction of the opposite direction of 1".
2 x 4
As this means "add 2 four (4) times",
2 x 4=2+2+2+2=8
(-2) x 4
As this means "add (-2) four (4) times",
(-2) x 4=(-2)+(-2)+(-2)+(-2)=-8
2 x (-4)
This means "add 2 negatively four (-4) times." "add (-4) times" means as its opposite direction "subtracting four (4) times." Thus
2 x (-4) =-2-2-2-2=-8
(-2) x (-4)
As this means "subtract (-2) four (4) times",
(-2) x (-4) = -(-2)-(-2)-(-2)-(-2) = 2+2+2+2=8
[Additioin]
Mystery of "1/(-1) = (-1)/1 =-1"
Below is somewhat distorted interpretation, so never believe it.
As 1 is greater than (-1), we can establish the equation: "Great / little = little / great" Why?
By the way, I don't know how to perfectly explain it. Nonetheless, I always use it "as it is."
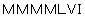 x
x 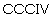 might not be easy due to their difficulty in notation.
might not be easy due to their difficulty in notation.

