For example, take the picture below:
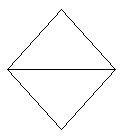
Below is the illustration when you successfully draw the unicursal figure.


[Rule of how to draw a unicursal figure]
To draw a unicursal figure is defined by tracing all the part of the closed loop forming some kind of picture without taking a pen apart from a sheet of paper on which the picture is drawn. Additionally, you cannot retrace the line already traced.
For example, take the picture below:

Below is the illustration when you successfully draw the unicursal figure.

[When was a unicursal figure successfully drawn in the world?]
I cannot tell when a unicursal figure was successfully drawn in the world because not having any technical book, but I can tell that it was done in Europe.
The problem was as follows:
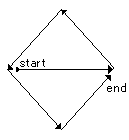
There are two islands in the center of a river, and bridges are thrown between the two islands as well as between lands and islands.
Now, you must cross every bridge, but must not recross any.
In the long run, this problem was found to change into the problem, that is, whether or not you can draw the unicursal figure below.
In addition, this problem was found out not to be solvable.
[Judgment of successfully drawing the unicursal figure]
Count the number of edges at a point into which such edges merge, and repeat this with all the points of the picture.
(1) If every point has an even number, then you can draw with one stroke while starting with any point you like
(2) If two points have odd numbers, then you can when starting with either point having odd number; and
(3) If not less than four points have odd numbers, then you cannot.
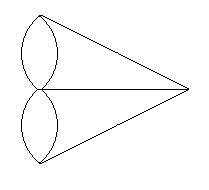
For example, when you count the numbers of edges with every point on the picture above:
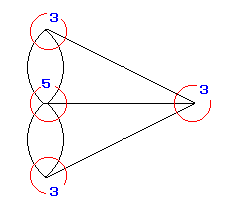
As shown in the illustration above, you cannot because four points have odd numbers.
[Principle]
Let us think with each point. If you are drawing a closed-loop picture, you never fail to have two edges, one of which goes to the point and the other of which comes from the same point. Thus, every point has an even number, then you can draw with one stroke. If there is any point having an odd number, then you should chose the point as a starting point. However, if there are not less than four points having odd numbers, then you cannot while starting with any point having an odd number because too many edges go to points.
NOTE: The points having odd numbers will never be odd in number such as 1 or 3.
|
Japanese sites Mathematical Formulas| Kodawari House| Pinpoint StreetView| Excel VBA Techniques| Excel Formula Analysis| |