I hit upon the following method of calculation during my sophomore year of college when I was working on the representation of algebraic equations.
When I taught this method to first-year students at a senior high school where I was working as a trainee teacher during my senior year of college, they were impressed, and today, this method appears to be widely used.
Let us carry out the following calculations by using this method.
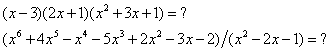
Solution
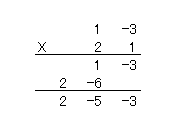
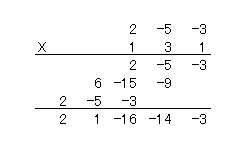
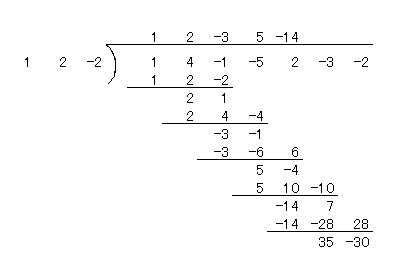
Therefore,
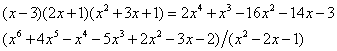
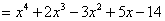, and the remainder is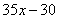 
This method can be interpreted as one that involves carry-free addition, subtraction, multiplication, and division.
Let's consider the recurring decimal. For example, for calculating 3/11, we have:
which results in 3/11 = 0.27272727···
We can use the above to calculate algebraic equations. For example, if we calculate 
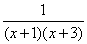
, we obtain:
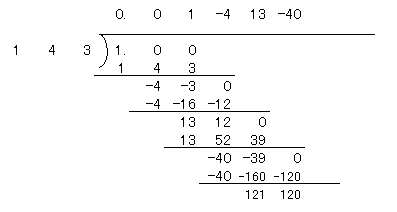
, and then
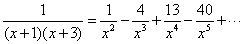
This result coincides with the Laurent expansion taught in undergraduate courses.
Here, even though we are not looking for the convergence of 
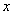, it converges for 
.
Further investigation may result in new applications.
|
Japanese sites Mathematical Formulas| Kodawari House| Pinpoint StreetView| Excel VBA Techniques| Excel Formula Analysis| |