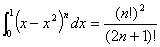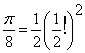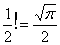Wallis / Integration of Parabola
[Integration of Parabola]
Wallis(1616-1703) had proven a math problem similar to integration in the "Arithmatica Infinitorum" published by Oxford. The problem was to seek the dimensions of the area surrounded by 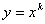 and
and  axis. The method used for proving was a mathematical solution making good use of the non-separability, mathematical induction and limit theory. As to the non-separability, kindly refer to Cavalieri's non-separability theory.
axis. The method used for proving was a mathematical solution making good use of the non-separability, mathematical induction and limit theory. As to the non-separability, kindly refer to Cavalieri's non-separability theory.
Although, this method may seem to be redundant with the researches of Roberval and Fermat, it is believed that Wallis had no knowledge of these two scholars.
In seeking the area of the rectangle 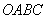 and the area ratio of the figure surrounded by parabola
and the area ratio of the figure surrounded by parabola 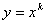 , we first define
, we first define 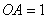 .
.

He divided the interval  into
into 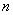 equal parts.
equal parts.
In the modern expression, the coordinates would be 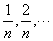 but because Wallis' method for proving is non-separability, he defined
coordinates as
but because Wallis' method for proving is non-separability, he defined
coordinates as 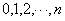 .
.
Draw a perpendicular line at each point and then seek the sum of the areas of the rectangles and the ratio of the areas surrounded by the parabola.

When  ,
,
Ratio
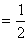
and when 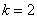 ,
,
he interpreted the case as the ratio of the areas of the triangle and rectangle.
Start with 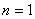 , and calculate in sequence.
, and calculate in sequence.


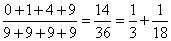
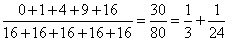
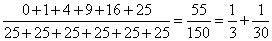
These ratios are always larger than 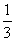 , and the differences would be
, and the differences would be 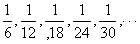 .
.
Since the denominator is increasing by 6 at a time, when 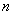 reaches an infinite value inductively, then the difference vanishes.
reaches an infinite value inductively, then the difference vanishes.
In other words, the ratio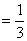 .
.
When 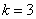 , calculate in the same manner as the case of
, calculate in the same manner as the case of 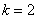 ,
,
then the ratio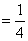 .
.
In the case of general 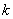 , he inductively got Ratio
, he inductively got Ratio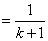 .
.

The rectangle 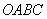 is divided by the function. By adding the upper area and the lower area of function, we get the area of a quadrangle. This is more a geometric interpretation, but when expressed in modern terms, it becomes
is divided by the function. By adding the upper area and the lower area of function, we get the area of a quadrangle. This is more a geometric interpretation, but when expressed in modern terms, it becomes
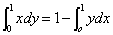 , and Wallis named it an interpolation.
, and Wallis named it an interpolation.
Using this equation, he calculated the integration of the cases where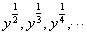 .
.
By generalizing further, he found that when 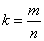 , then the Ratio
, then the Ratio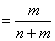 .
.
[Expansion of Factorial]
Wallis knew that the area of a semicircle is 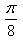 but he went on to study a method that uses non-separability to seek the area of semicircle. In modern expression, it becomes
but he went on to study a method that uses non-separability to seek the area of semicircle. In modern expression, it becomes 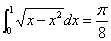 .
.
However, Wallis could not find the method that could calculate directory.Therefore, he used as a natural number and calculated as follows:
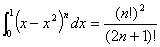
He also presumed that this expression could work out for fraction, he went forward and expressed the below equation:
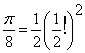
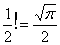
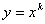 and
and  axis. The method used for proving was a mathematical solution making good use of the non-separability, mathematical induction and limit theory. As to the non-separability, kindly refer to Cavalieri's non-separability theory.
axis. The method used for proving was a mathematical solution making good use of the non-separability, mathematical induction and limit theory. As to the non-separability, kindly refer to Cavalieri's non-separability theory.

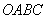 and the area ratio of the figure surrounded by parabola
and the area ratio of the figure surrounded by parabola 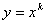 , we first define
, we first define 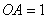 .
.
 into
into 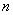 equal parts.
equal parts.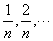 but because Wallis' method for proving is non-separability, he defined
coordinates as
but because Wallis' method for proving is non-separability, he defined
coordinates as 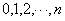 .
.
 ,
,
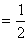
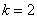 ,
, , and calculate in sequence.
, and calculate in sequence.

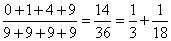
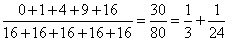
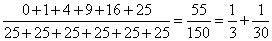
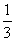 , and the differences would be
, and the differences would be 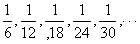 .
.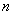 reaches an infinite value inductively, then the difference vanishes.
reaches an infinite value inductively, then the difference vanishes.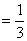 .
.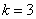 , calculate in the same manner as the case of
, calculate in the same manner as the case of 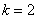 ,
, 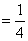 .
.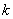 , he inductively got Ratio
, he inductively got Ratio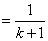 .
. is divided by the function. By adding the upper area and the lower area of function, we get the area of a quadrangle. This is more a geometric interpretation, but when expressed in modern terms, it becomes
is divided by the function. By adding the upper area and the lower area of function, we get the area of a quadrangle. This is more a geometric interpretation, but when expressed in modern terms, it becomes 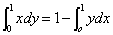 , and Wallis named it an interpolation.
, and Wallis named it an interpolation.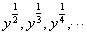 .
.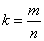 , then the Ratio
, then the Ratio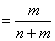 .
.
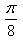 but he went on to study a method that uses non-separability to seek the area of semicircle. In modern expression, it becomes
but he went on to study a method that uses non-separability to seek the area of semicircle. In modern expression, it becomes 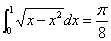 .
.