

Napier, Briggs / logarithm
Napier(1550-1617) was a squire in Scotland and was not an expert in mathematics. At this time, it was known that the multiplications and divisions of exponential multiplier sequences were corresponding to the exponential sums and differences. However, this exponential multiplier sequences could not be used as a calculation tool because the intervals between the sections of the exponential multiplier sequences were simply too big. Around 1594, Napier hit on the idea of the concept of logarithm as a means to fill the large intervals of exponential multiplier sequences and had published "Mirifici logarithmorum canonis constructio" in 1614.
The principle of the logarithm was geometrical.
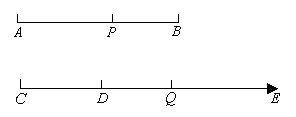
First, a line segment  and a half line
and a half line 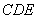 are drawn. The point
are drawn. The point 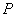 starts from
starts from  and moves to point
and moves to point  at a velocity decreasing in proportion to the distance from
at a velocity decreasing in proportion to the distance from 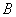 . At the same time, the point
. At the same time, the point 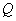 starts from
starts from 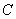 and moves over
and moves over 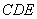 at a uniform velocity. It was defined that "the distance
at a uniform velocity. It was defined that "the distance 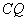 is the log of the distance
is the log of the distance  ".
".
Because the decimal fractions of the decadal system was undeveloped at
that point in time, in order to avoid decimal fractions, the below expression
was formulated where " would be the Napier log of
would be the Napier log of 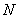 ".
".
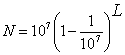
In 1615, Briggs(1561-1630), Professor of geometry at Oxford, started to modify logarithmic law jointly with Napier and had completed common logarithm referred to as exponential in decimal.
After the death of Napier, Briggs made a logarithmic table and this table had greatly contributed to the multiplications and divisions in astronomic calculations. In that era, the discovery of logarithm was not regarded as the major mathematical matter of concern.
For instance, in the case of calculating 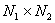 , the way to use this table is to find
, the way to use this table is to find 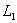 corresponding to
corresponding to 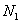 and then look for
and then look for 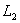 corresponding to
corresponding to  in the entries of logarithmic table. The next step is to calculate
in the entries of logarithmic table. The next step is to calculate  . The sequence is followed by the work of finding
. The sequence is followed by the work of finding  corresponding to
corresponding to  in the entries of logarithmic table.
in the entries of logarithmic table.

 and a half line
and a half line 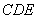 are drawn. The point
are drawn. The point 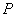 starts from
starts from  and moves to point
and moves to point  at a velocity decreasing in proportion to the distance from
at a velocity decreasing in proportion to the distance from 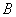 . At the same time, the point
. At the same time, the point 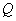 starts from
starts from 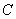 and moves over
and moves over 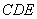 at a uniform velocity. It was defined that "the distance
at a uniform velocity. It was defined that "the distance 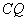 is the log of the distance
is the log of the distance  ".
".


 would be the Napier log of
would be the Napier log of 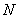 ".
".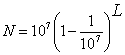
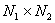 , the way to use this table is to find
, the way to use this table is to find 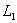 corresponding to
corresponding to 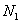 and then look for
and then look for 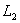 corresponding to
corresponding to  in the entries of logarithmic table. The next step is to calculate
in the entries of logarithmic table. The next step is to calculate  . The sequence is followed by the work of finding
. The sequence is followed by the work of finding  corresponding to
corresponding to  in the entries of logarithmic table.
in the entries of logarithmic table.